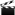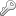.
O P E N A C C E S S S O U R C E : Cell Press_Trends in Genetics
Aging entails an irreversible deceleration of physiological processes, altered metabolic activities, and a decline of the integrity of tissues, organs, and organ systems. The accumulation of alterations in the genetic and epigenetic spaces has been proposed as an explanation for aging. They result, at least in part,from DNA replication and chromosome segregation errors due to cell division during development, growth, renewal, and repair. Such deleterious alterations,including epigenetic drift, irreversibly accumulate in a stepwise, ratchet-like manner and reduce cellular fitness, similar to the process known as Muller’s ratchet. Here, we revisit the Muller’s ratchet principle applied to the aging of somatic cell populations and discuss the implications for understanding the origins of senescence, frailty, and morbidity.
Genetic and Epigenetic Somatic Variation as a Catalyst of Senescence and Aging
Aging entails an irreversible deceleration of physiological processes at the cellular level. It is accompanied by altered metabolic activity and a decline of the integrity of tissues, organs, and organ systems [1]. The cumulative impact of such deterioration is paralleled by functional declineand affects viability and reproduction. In principle, senescence and aging accompany differentiation, development, and growth, a set of processes that is initiated with the formation of the zygote (see Glossary)[2]. Thus, aging represents cumulative and deteriorative processes impacting the architecture, development, and growth of cell populations and individuals at all levels of the genotype–epigenetic–phenotype (G-E-P) space with the passage of time [3].From a gerontological perspective, however, aging is primarily restricted to post-reproductive stages, during which many organs and organ systems of individuals show both physiological and functional decline, collectively termed frailty. Specifically, cellular frailty encompasses dynamic and stochastic processes of accumulation of deficits at the subcellular and cellular levels[4,5]. Such independent or cumulative system-wide decline of physical and physiological factors contributes to the manifestation of frailty of the whole organism [6]. Thus, senescence, frailty, and morbidities may share common origins [7]. For clarity, although aging and senescence are often used interchangeably, we equate senescence with irreversible cessation of the mitotic activities of normal somatic cells [8]. Similarly, because somatic mutations potentially start accumulating from the first mitotic division of the zygote, we define ‘aging as all possible changes between conception and death’[2].
Several theories have been proposed to explain aging. One is based on the accumulation and amplification of inherited and acquired (somatic) genetic variants [mutation accumulation(MA) theory][9–11]. Another theory, by Muller [12], emphasized the negative and cumulative effects of such accumulating mutations or mutation load (ML) that impose a burden, ‘measured in terms of reduced fitness, but felt in terms of death, sterility, illness, pain and frustration’[13]. Clearly, both MA and ML result from sequential cell division generated by growth and development, renewal, and repair, which inevitably engenders DNA replication and chromosome segregation errors. Thus, the burden of somatic variants increases with age. The MA and ML theories, subtleties aside, converge on the adverse effects that genomic variation exerts on somatic and reproductive tissues and, ultimately, on health and longevity. The accumulation of somatic mutations is initiated at the first cell division and increases through successive mitotic divisions. Therefore, we assume that 'biological aging' is initiated at the time of zygote formation, a view consistent with demographic and genetic principles [14,15]. The MA process is a gradual and ubiquitous feature among all differentiating cell lineages in an organism [16] and is comparable with what happens in asexual or clonally reproducing organisms [17]. Each additional de novo deleterious variant [18] may have both cumulative and multiplicative effects at various levels of organismic organization –genome instability,molecular heterogeneity, altered gene expression, impaired intercellular communication, tissue disintegration, vulnerability to stress, etc. –which may influence both fitness [19] and healthspan [20,21].
Genetic variants accumulate at a rate of roughly one or two per cell division during embryogenesis[22]. Thus, cell division over decades entails the accumulation of billions of variants in every tissue and organ. The extent of this phenomenon can be appraised in an adult human, who comprises about 37 trillion cells [23] all of which stem from a single cell, the zygote [24]. The Muller’s ratchet principle suggests that genomic alterations accumulate in a stepwise, ratchet-like manner (Figure 1A), especially in asexual lineages [25,26], and in non recombining genomes or genomic regions. This process eventually leads to an increase in the average number of unfavorable variants present in the genome of each cell [25], entailing a gradual decline of fitness of individual cells and tissues. Figure 1B shows a modified Muller plot [17], which is a convenient way to represent this time-dependent process.

Accumulation of (Epi)Genetic Alterations in Cell Division during Development and Subsequent Stages of Life. (A) Production of cell lineages with different mutation loads. (B) Modified Muller plot describing the same process in time. It combines information on the succession of different genotypes/phenotypes (horizontal axis) and their abundances (vertical axis). Different cell genotypes are represented by areas with different colors. Each genotype originates by mutation of the parental one.
The epigenome also changes across cell generations [27]. For instance, aging mammalian cells display generalized DNA hypomethylation, primarily in DNA repeats, and local hypermethylation concentrated at promoters. This epigenetic drift results from incomplete restoration of normal epigenetic patterns after DNA replication or repair. It is known that epigenetic marks in twins are similar during infancy but change with age, impacting gene expression. The impact of epigenetic variation on aging needs to be considered along with that of genetic alterations because of its higher frequency and because epigenetic drift causes departures from the norm by manyorders of magnitude [28,29]. Losses and gains of methylation during replicative senescence are similar to those observed in cancer. Thus, the epigenome of senescent cells might promote malignancy, if such cells escape the proliferative barrier [30,31].
From the perspective of the G-E-P map [32,33], it is reasonable to suggest that the lifelong accumulation of genetic and epigenetic changes in cells and tissues alters physiological homeostasis and ultimately leads or at least contributes to aging [34]. Along with the Muller’s ratchet principle applied to aging, we postulate that the frailty phenotypes that arise with age are a function of the load of harmful genetic and epigenetic variants carried by the individual and its components. In short, in a demographic sense, the degree of frailty is linked to the (epi)mutational burden carried by the various cell lineages in relation to their age and stage of development [12,35].
The mechanisms by which mutations of clonal origin influence aging and disease remain obscure[36]. Thus, ideas from evolutionary genetics can be extended to gain insights on some of the en-during questions in aging and human health. Although the (epi)genetic basis of the relationship among cellular aging, cancer, and Muller’s ratchet has been suggested by various investigators,a detailed population and demographic genetic analysis of this phenomenon in terms of frailty and morbidity is still required. Thus, we have extended here the Muller’s ratchet principle to the biological aging process in somatic tissues [15] and discuss its implications for understanding the origins of frailty, senescence, and morbidity.
Muller’s Ratchet Model Applied to Aging
The Muller’s ratchet in relation to cell proliferation and tissue formation can be modeled as a Moran birth–death demographic process, which describes the stochastic birth and death of individuals in finite populations. Accordingly, de novo genetic variants, with varying degrees of deleterious effects, differentially accumulate among cells and tissues during the course of development. The Moran model allows us to explore the health ('viability fitness') consequences of such mutation-bearing clonal lineages. As the individual is a mosaic of cells and lineages of cell populations with different sets of somatic variants, heterogeneity among them also increases[37], leading to spatial structuration [38]. By extension, we postulate that each specific cell population could show a range of viability fitness in adult tissues [39], which might translate into the existence of ‘ageotypes’ at the population level [40]. Although this assumption is reasonable, experimental evidence for fitness differentials among cell lineages in relation to the accumulation of genetic variants in humans remains to be established [41]. Recent studies, however, point toward such a possibility [42,43].The formal principles behind the model can be outlined as follows. We can reasonably consider that the cell is the unit of differentiation, development, growth, and regeneration [44,45] and that tissues and organs are mosaics of cells carrying different mutation loads. Our model starts from a single ‘stem’cell through differentiation, development, and growth into a mature individual.Thus, a population of N cells at any given time point results from a single cell. The model also en-compasses gradual physiological decline. Each cell can be characterized by three time-dependent parameters, its rate of division (b), its functionality or healthiness (f), and its death rate (d), which depend on the number and impact of (epi)genetic variants that the cell has accumulated over its entire life. However, keep in mind that mutations can occur not only through cell division but also in a replication independent manner (Box 1).

Typical Patterns of Aging Obtained by Mathematical Simulation. (A) Normal aging was simulated assuming N = 100, b = 0.1, d = 0.05, and a pergenome mutation rate = 1. During the growth phase (b NN d), cell death d is small and cell division prevails, leading to an increase of N (green rectangle). Afterwards, the system reaches a stationary phase (yellow rectangle). When b ≥ d, a dead cell is removed and replaced by cell division. This process entails the accumulation of deleterious (epi)genetic variants (thus, b decreases and d increases). After T b = d (vertical red arrow), the empty space left by a dead cell is not be filled by a new one and the size of the cell population decreases (decline phase; orange). The functionality of the tissue, coded in red, in the topmost panel decreases with time. (B) In the case of fast aging (i.e., d = 0.06), the span of the stationary phase is shortened, leading to an earlier decline of the cell population.
These three parameters may be affected by developmental stages and the environment. If we consider the negative effects of accumulating deleterious variants [46,47], f and bare supposed to decrease and d to increase with time. Assuming that f0 is the parameter at birth, the effect of mutation accumulation is given by f=f0(1 –δf1)(1 –δf2)…(1 –δfi).(1 –δfn), where δfi is the reduction in the functionality caused by the ith mutation. A similar reasoning applies to the other parameters.
After a lag phase, the number of cells (N) rapidly increases up to a value K, representing the adult population size (growth phase). The cell population can maintain this size K when cells are healthy enough (so that b Nd; stationary phase). As aging proceeds, the cell population steadily becomes unhealthy and N declines (i.e., K cannot be maintained any longer). This decline phase appears when the cell birth rate (b) becomes smaller than the population death rate (d). Throughout the whole process, the average of the number of accumulated mutations per cell (Save) increases, especially rapidly in the growth phase. Box 1 provides further details on the principles and consequences of the model.
What the Model Says
The growth of a tissue from pluripotent stem cells to its adult size relies on a large number of cell divisions, which are associated with the accumulation of numerous potentially deleterious variants among cells. Damage at the molecular level includes both benign and pathogenic somatic variants that coexist with an array of inherited variants [48]. The latter (constitutional) ones exert in most cases more powerful effects relative to somatic variants as they have been present since conception and affect all of the cells of the individual. Somatic variants, in concert with constitutional variants, will influence health, cancer, and the course of hereditary diseases [49,50].
Our model and simulations suggest that the cell birth and death rates are crucial to determine the pattern of aging. In tissues like the brain, where cells experience a relatively low death rate, genetic variants mainly accumulate during early stages of development, after which replication-independent mutations are the main contributors. By contrast, in a tissue with a relatively high rate of cell turnover, (epi)genetic variants keep accumulating all of the way, even during the stationary phase, so aging proceeds during maturity. These differences are illustrated in Box 1 (see Figure I in Box 1), which show typical patterns of aging in normal tissues together with an abnormal case.In both cases, the cell population size rapidly increases up to the adult size, arbitrarily set at K=100. Through the growth phase, the average number of accumulated somatic variants, Save,in-creases, resulting in a decline of cell healthiness [f; represented by the yellow-to-orange transition(see Figure I in Box 1)]. The major difference between the two cases displayed is the death rate(d). In the normal-aging case, with d=0.5b (see Figure IA in Box 1), turnover of cells occurs quite often, leading to the accumulation of new somatic variants after maturity (i.e., stationary phase). In the normal-aging case, Save keeps increasing in the stationary phase, resulting in further decreases of band f and an increase of d. Eventually, d exceeds b. After this time point (denoted by TB=D), the system enters the decline phase. In progeroid syndromes, such as Hutchinson–Gilford syndrome[51], TB=Dis small. In Box 1 we show a simulation where the curves cross at around 25 years of age,as in the case of progeria patients (see Figure IB in Box 1)[52]. In sum, a cell population can maintain a stable size when b > d, but once d exceeds b the population size starts declining and cells are no longer able to fill all of the empty space left by dead ones. In this decline phase, the aging process is accelerated, accompanied by a decline in cell population size. Thus, according to our simple model,band dare key factors determining the pattern of aging among cell populations. There is some experimental data supporting this concept in telomere-dysfunctional mice. Both slowing of cell birthrates and increases in cell death rates aggravate organism aging in the context of short telomeres [53,54].
.../...
.
Edited by Engadin, 10 May 2020 - 05:21 PM.